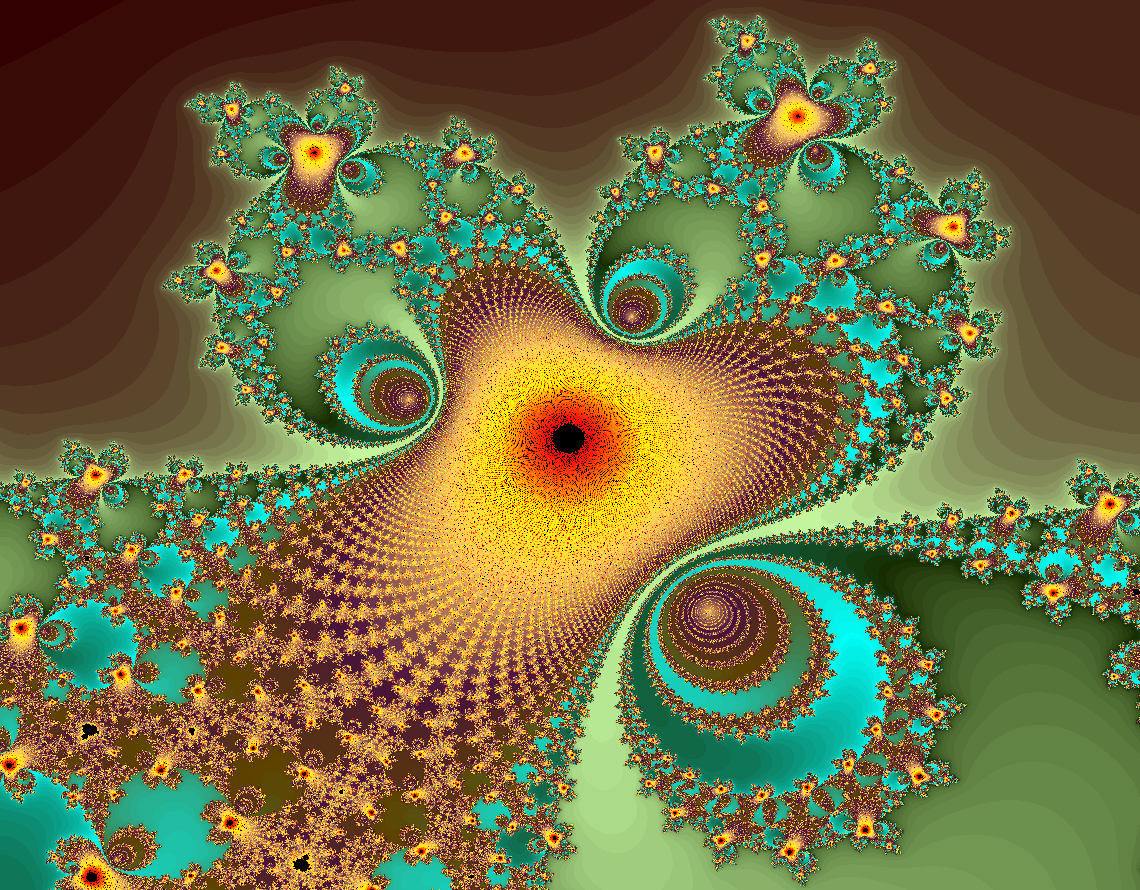
WHAT IS A FRACTAL?
When you study calculus, you study objects that are smooth. Smooth objects have tangents. If it is 1-dimensional, a smooth object has a tangent line at every point. If it is 2-dimensional, it has a tangent plane at every point. Fractals are not smooth. They have a simple property which makes it almost impossible for them to have tangents. When you look at a fractal through a microscope, it looks the same as if it were not magnified. In other words, every irregularity is repeated again and again at a smaller scale every time you increase the magnification. In the fractals below, you can see smaller and smaller images of the larger picture.

If you think about the definition of the tangent line that you learned in calculus, you might remember that the tangent line is defined as the limit of secant lines. These secant lines are drawn through two points and the tangent line is the limit when the two points approach each other. In other words, the slopes of the secant lines must stabilize when the two points approach closely and the limiting value is the slope of the tangent line. Imagine what this means. It means that, as we magnify more and more, the figure looks more and more linear. If it is 1-dimensional, linear means "like a line." If it is 2-dimensional, linear means "like a plane." This is not what happens in a fractal where every bump and irregularity repeats itself as you magnify.
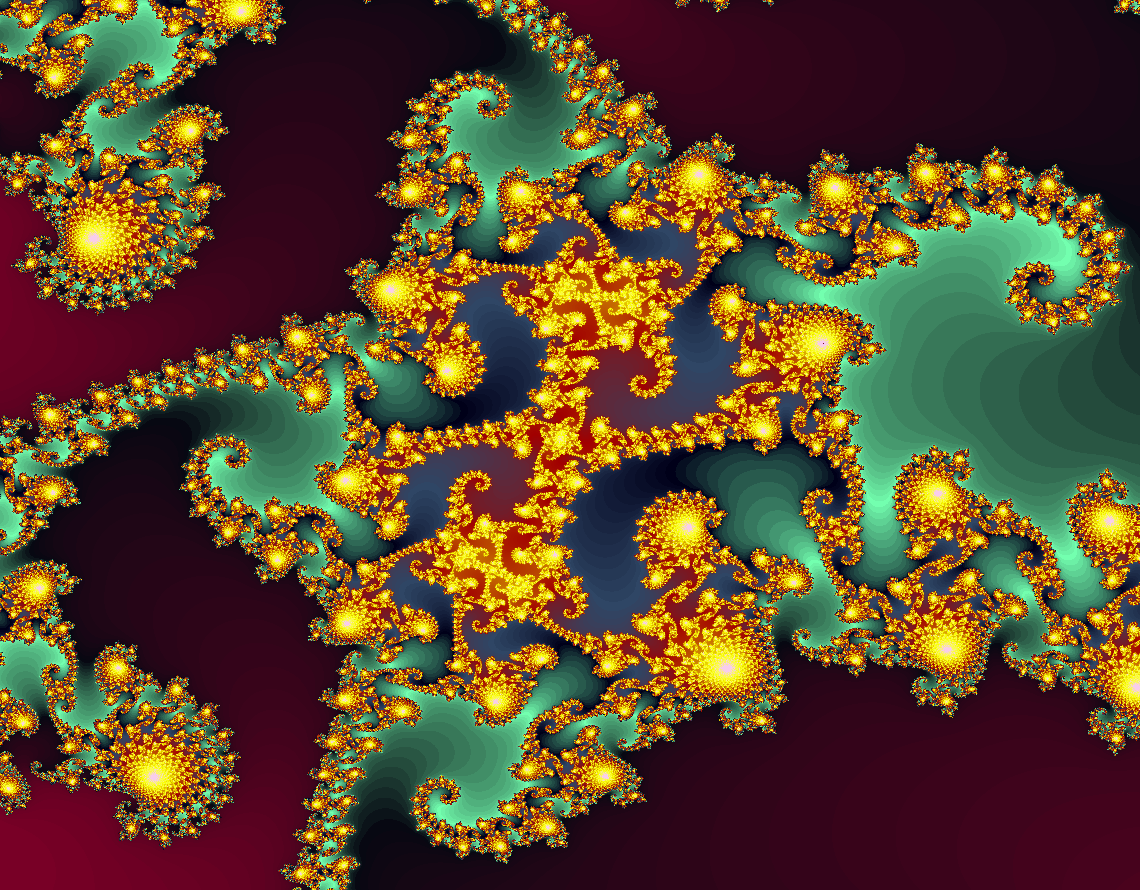
There is a sense in which the smooth objects of calculus are always idealizations. This means that they are simpler models of nature which achieve their power and strength precisely because they ignore some of the inherent complications in order to gain computability. It is an art to decide which complications can safely be ignored and still retain the essential properties of the situation. Fractals do not ignore things in the same way. They are full of noise and complication, repeated infinitely often as you change the scale, but, as you can see from these pictures, they have another kind of regularity inherent in the very nature of this repetition.